IPD in Dynamic Markets
Modeling Dynamic Oligopolistic Structures
This was a one-day project with two of my peers that aimed to advance the Iterated Prisoner’s Dilemma (IPD) model to reflect dynamic market conditions, providing a realistic representation of strategic adaptations in oligopolistic markets.
Oligopolies: Market structures with a small number of players, where each player's decisions significantly impact the entire market.
Iterated Prisoner’s Dilemma: A game theory model used to study cooperative and competitive behaviors among rational decision-makers. Traditional models use static payoff matrices, which do not accurately reflect changing market conditions.
Background
Previous IPD research has focused on constant payoff matrices, which do not account for the dynamic nature of real-world markets.
Problem
Develop a dynamic IPD model that adjusts payoff matrices based on game outcomes, providing a more accurate representation of oligopolistic market conditions.
Solution
Strategy Definition: Use general IPD strategies: Cooperator (always cooperates), Defector (always defects), Random (defects or cooperates with equal probability), and Tit for Tat (mimics opponent's previous move).
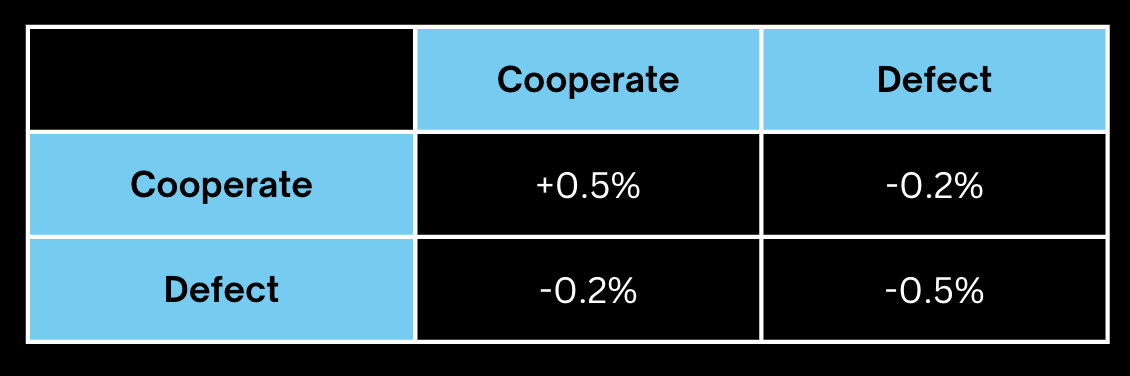
Payoff Matrix Development: Create a symmetric payoff matrix reflecting cooperative and competitive interactions, with payoffs changing based on market outcomes.
Simulation Setup: Run a round-robin tournament with each strategy pairing, iterating multiple rounds to simulate market conditions and strategic adaptations. Each player competes with every other player 100 times.
Methodology
Initial Payoff Matrix
Payoff Percent Change Matrix
Results & Discussion
Exploitation & Defense: Tit for Tat (adaptive and reciprocal) effectively defends against exploitation, particularly in Defector vs. Cooperator interactions.
Stability of Cooperation: Mutual cooperation strategies, such as Cooperator vs. Cooperator and Tit for Tat vs. Tit for Tat, yield the highest payoffs.
Importance of Adaptability: Tit for Tat's ability to adapt to opponents’ strategies maximizes payoffs, performing well against both Cooperators and Defectors.
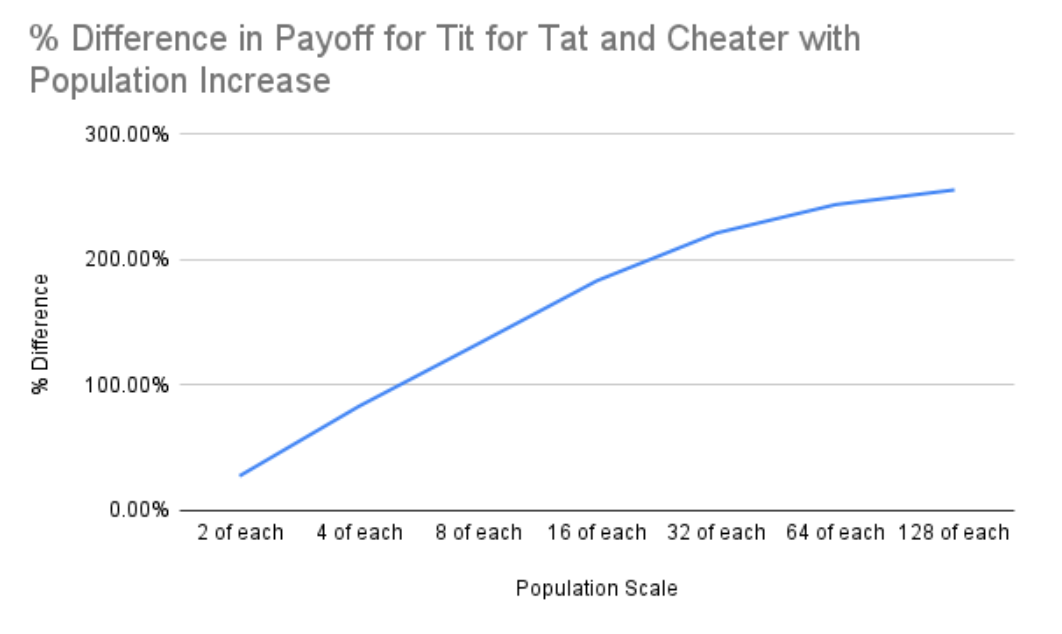
Increasing efficacy of the Tit for Tat strategy as compared to the Cheater strategy in terms of percentage difference in payoffs, as the population scales up.
Clear and consistent trend: as the population increases, Tit for Tat not only retains but also enhances its effectiveness relative to the non-adaptive Cheater strategy.
Conclusion
The effectiveness of Tit for Tat in maximizing payoff relative to the Cooperator while also minimizing the relative payoff of the Defector indicates that a mixed strategy is most effective in environments where some parties may be induced to cheat. Tit for Tat’s ability to consistently bring greater returns than Cooperator suggests that oligopolistic cartels become increasingly ineffective for faithful members as individual parties continue to cheat. The Defector’s decreased relative payoff is also indicative of how continued defectors do worse as the size of the cartel increases, possibly due to the fact that their continuous defection hurts the market greater when it is larger. These results are reflected in historic oligopolistic structures, whose cartels often collapse due to repeated cheating without greater incentives to collude.